Gruebler Paradoxes

There are some examples of paradoxes which disobey the Gruebler’s criterion due to their unique geometry. The designer needs to be alert to those possible inconsistencies. As the Gruebler’s criterion pays no attention to link sizes  or shapes, it can  give misleading  results in the face of unique  geometric  configurations.

For example, Fig. (a) shows a structure (DOF = 0) with the ternary links of arbitrary shape. This link arrangement is sometimes called the “E-quintet”, because of its resemblance to a capital E and the fact that it has five links including the ground.

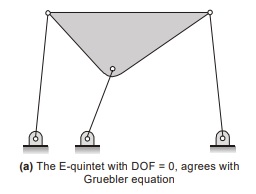
Fig. (b) shows the same E-quintet with the ternary links straight and parallel and with equispaced nodes. The three binary links are also equal in length. With this very unique geometry, you can see that it will move despite Gruebler’s criterion.

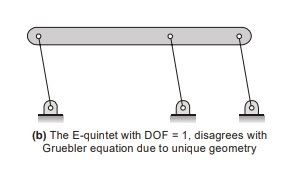
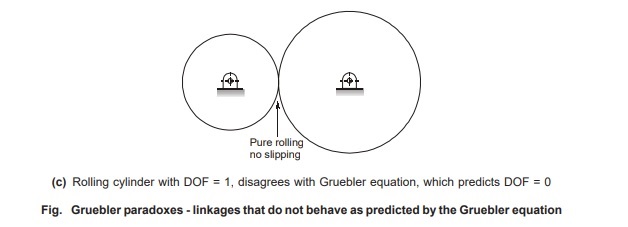
Fig. (c) shows a very common mechanism which also disobeys Gruebler’s criterion. The joint between the two wheels can be postulated to allow no slip, provided that sufficient friction is available. If no slip occurs, then this is one DOF which allows only relative angular motion (Δθ) between the wheels.

<< Previous | Next >>
Must Read: What is the Theory of Machines?