Mason Gain Formula

The relationship between an input variable and an output variable of a signal flow graph is given by the net gain between the input and output nodes and is known as the overall gain of the system.
However, It is possible to simplify signal flow graphs in a manner similar to that of block diagram reduction, but the task of solving for the input output relations by algebraic manipulates could be quite cumbersome.
Fortunately, it is also possible and much less time consuming to write down the input-output relationship by inspection from the original signal flow graph by using Mason’s gain formula.

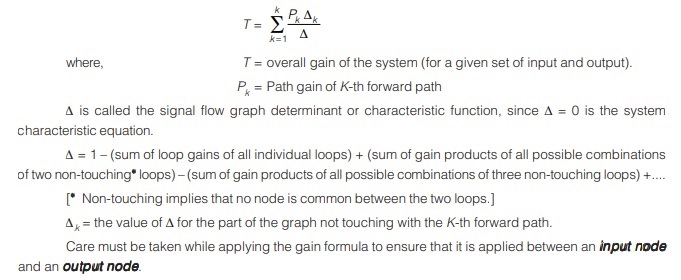
Mason’s gain formula for the determination of the overall system gain is given by

Feedback and Non-Feedback Systems

Feedback as a means of automatic regulation and control is inherent in nature and can be noticed in many physical, biological and soft systems. For example, the body temperature of any living being is automatically regulated through a process which is essentially a feedback process, only it is far more complex than the diagram of fig. (b)

Key benefits of feedback are enumerated below:

• The controlled variable accurately follows the desired value.
• Effect on the controlled variable of external disturbances other than those associated with the feedback sensor are greatly reduced.
• Effect of variation in controller and process parameters (the forward path) on system performance is reduced to acceptable levels. These variations occur due to wear, aging, environmental changes etc. Feedback in the control loop allows accurate control of the output even when process or controlled plant parameters are not known accurately.
• Feedback in a control system greatly improves the speed of its response.