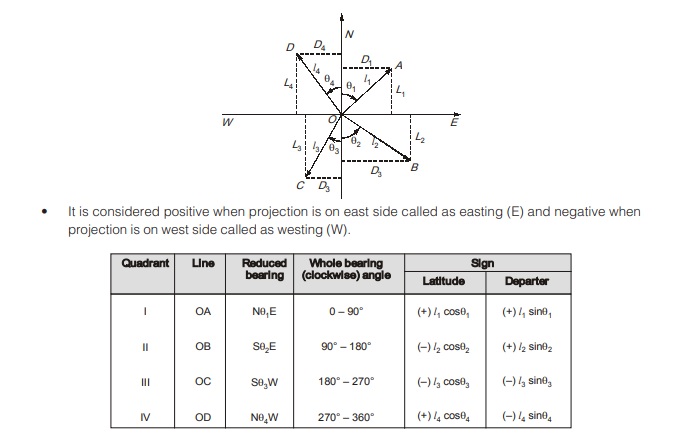
COMPUTATION OF LATITUDE AND DEPARTURE

• The latitude (L) of a line is the orthographic projection of the line on the N-S axis representing the Meridian.
• It is considered positive when the projection of the line is on the north called as northing (N) and negative when on south side called a southing (s).
• The departure (D) of a line is the orthographic projection of the line on the axis perpendicular to the Meridian i.e. E-W axis.

Note that in the expression for

Latitude = l cos0,

Departure = l sin0

Where 0 = angle measured from N – S axis.

if, in place of quadrant bearing angle, we use whole circle bearing, the value so obtained will have the necessary sign. For example, if the line OC ha 0₃ = 30°, the whole circle bearing will be 210°.

Hence

latitude = l3 cos210° = – 0.866 l3(i.e. – ve)
departure = l3 sin210° = – 0.5 l3 (i.e. – ve)

Consecutive and Independent Co-ordinate

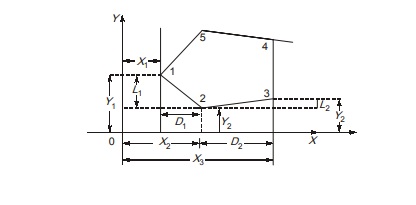
Consecutive Co-ordinates

• Co-ordinates of end point of a line w.r.t to its initial point are called consecutive co-ordinate or dependent co-ordinates of the end point of the line.
• Consecutive co-ordinate of end point 2 of line 1 – 2 will be – L₁ and D₁.

Independent co-ordinates

• Co-ordinates of a point w.r.t to a common origin are called independent co-ordinates.
• Independent co-ordinate of point 1 = y₁ and x1.
• Independent co-ordinate of point 2 = y₂ and x2.

NOTE:   

x2 = x1 + D1
y2 = y1 – L1
x3 = x1 + D1 + D2
y3 = y1 – L1 + L2

• Total latitude of any point (say point no. 3) = Total latitude of starting point + algebraic sum of the all latitude of all lines upto that point 3.
• Total departure of any point (say point number 3) = Total departure of starting point + algebraic sum of the departures of all lines upto that point 3.

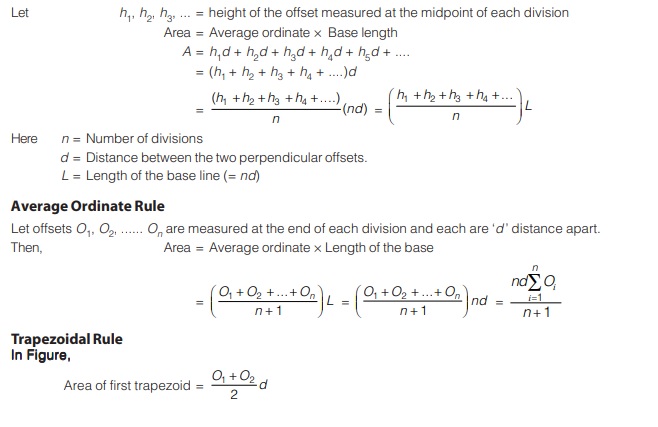
Mid-ordinate Rule

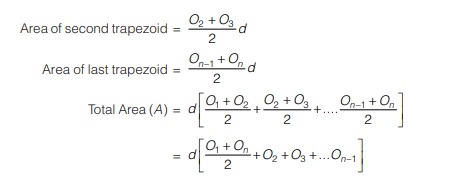
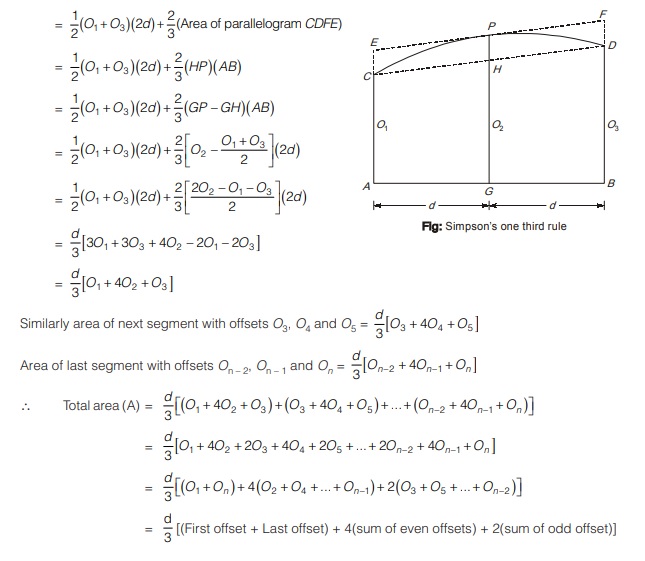
Simpson’s One Third Rule

Here it is assumed that the boundaries between the two extreme ends of three consecutive offsets is parabolic and the axis of parabola is parallel to the offsets.

Now, Area of figure = Area of trap. ABDC + Area of parabolic portion CHDPC

Note:

In order to apply Simpson’s one-third rule, the areas must be divided in even number i.e., number of offsets must be odd i.e., n term in the last offset ‘O’n should be odd. If offsets are even in number, then in this case average of offsets is taken between any two adjacent offsets and hence the number of offsets become odd.

<< Previous | Next >>
Must Read: What is Surveying?